Introduction
The first description of the turbulence cascade came with the intuitive scheme of Richardson. This was later formalized by the self-similarity hypothesis ([Kolmogorov, A. N, 1941][1]). The first attempt to provide a quantitative description of the Richardson cascade was made by [Yaglom, A. M, 1966][2] and [Gurvich, A. S., & Yaglom, A. M, 1967][3].
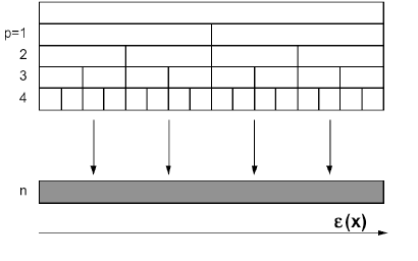
 Information[4]
Information[4]Different discrete and continuous cascade models have been introduced to describe intermittent fluxes. A first family of models is composed of discrete models, for which the scale ratio between a structure and the daughter structure is a discrete integer. Due to their discrete nature, these models are not realistic but have been introduced for their simplicity and ability to reproduce experimental
intermittency. These models include the :
β-model
α-model
p-model
...., etc.





