Extremes

Lesson

Introduction
Theory
Extreme value theory
Multifractal phase transition
- Introduction
- Bare and Dressed quantities
- First order phase transition
- Empirical power law estimation
To go further
Exercises
Conclusion

Empirical power law estimation

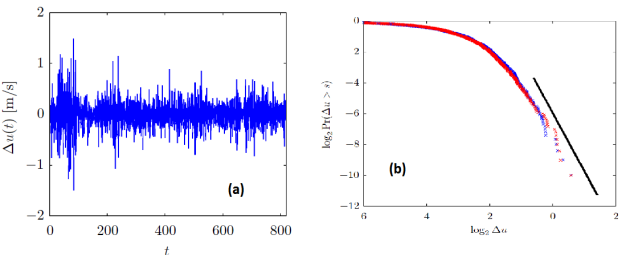
To quantify the behavior of the extremes of a distribution is to try to t a power law of fit the heavy tail(s). We can estimate the exponent by taking the linear regression of the same probabilities versus however in a log-log plot. In the following figure we estimate the tail of the distribution of the wind velocity increment, the corresponding divergent moment is .

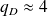(a): A ten-minute time-series of the u-component velocity increments, (b):The exceedance probability of the positive (red) and negative (blue) velocity The slope of the tail corresponding to the power law. Fitton, 2013 | Information^[1]

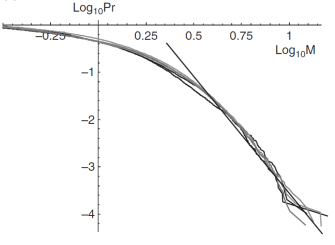
(a): A ten-minute time-series of the u-component velocity increments, (b):The exceedance probability of the positive (red) and negative (blue) velocity The slope of the tail corresponding to the power law. Fitton, 2013 | Information^[1]

The second example shows the predicted power-law behaviour for nondimensional raindrop distributions for which

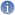Probability distributions of raindrop volumes nondimensionalized by dividing by the mean mass The reference line has absolute slope qD = 5, Lovejoy and Schertzer 2013 | Information^[2]

Fitton, G. (2013). Multifractal analysis and simulation of wind energy fluctuations (Doctoral dissertation, Université Paris-Est).
Lovejoy, S., & Schertzer, D. (2013). The weather and climate: emergent laws and multifractal cascades. Cambridge University Press.

Homepage