Source Of Complexity

Lesson

Classification of PDEs

The partial differential equations in general are classified into three categories: (a) elliptic, (b): parabolic, (c): hyperbolic. In geophysics field, most of the governing equations are second order partial differential equations. Lets give some PDEs corresponding to the above classification

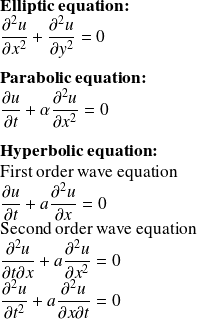
$\text{\textbf{Elliptic equation:}}\\ \frac{\partial^2 u}{\partial x^2} + \frac{\partial^2 u}{\partial y^2} = 0\\ \quad\\ \text{\textbf{Parabolic equation:}}\\ \frac{\partial u}{\partial t} + \alpha \frac{\partial^2 u}{\partial x^2} = 0\\ \quad\\ \text{\textbf{Hyperbolic equation:}}\\ \text{First order wave equation}\\ \frac{\partial u}{\partial t} + a \frac{\partial^2 u}{\partial x} = 0\\ \text{Second order wave equation}\\ \frac{\partial^2 u}{\partial t \partial x} + a \frac{\partial^2 u}{\partial x^2} = 0\\ \frac{\partial^2 u}{\partial t^2} + a \frac{\partial^2 u}{\partial x \partial t} = 0$

The equations describing the evolution of complex systems are of mixed type. The term characterizing the diffusion of concentrations or species can be represented by an elliptic PDEs, the advection or convection (heat transfer) can be represented by a hyperbolic PDEs.

Homepage